Exercises

Author(s)

David M. Lane

Prerequisites

All material presented in the ANOVA Chapter

Selected answers

  1. What is the null hypothesis tested by analysis of variance?
  2. What are the assumptions of between-subjects analysis of variance?
  3. What is a between-subjects variable?
  4. Why not just compute t-tests among all pairs of means instead computing an analysis of variance?
  5. What is the difference between "N" and "n"?
  6. How is it that estimates of variance can be used to test a hypothesis about means?
  7. Explain why the variance of the sample means has to be multiplied by "n" in the computation of MSB.
  8. What kind of skew does the F distribution have?
  9. When do MSB and MSE estimate the same quantity?
  10. If an experiment is conducted with 6 conditions and 5 subjects in each condition, what are dfn and dfe?
  11. How is the shape of the F distribution affected by the degrees of freedom?
  12. What are the two components of the total sum of squares in a one-factor between-subjects design?
  13. How is the mean square computed from the sum of squares?
  14. An experimenter is interested in the effects of two independent variables on self esteem. What is better about conducting a factorial experiment than conducting two separate experiements, one for each independent variable?
  15. An experiment is conducted on the effect of age and treatment condition (experimental versus control) on reading speed. Which statistical term (main effect, simple effect, interaction, specific comparison) applies to each of the descriptions of effects.
    1. The effect of the treatment was larger for 15-year olds than it was for 5- or 10-year olds.
    2. Overall, subjects in the treatment condition performed faster than subjects in the control condition.
    3. The difference between the 10- and 15-year olds was significant under the treatment condition.
    4. The difference between the 15- year olds and the average of the 5- and 10-year olds was significant.
    5. As they grow older, children read faster.
  16. An A(3) x B(4) factorial design with 6 subjects in each group is analyzed. Give the source and degrees of freedom columns of the analysis of variance summary table.
  17. The following data are from a hypothetical study on the effects of age and time on scores on a test of reading comprehension. Compute the analysis of variance summary table.
    18.   12-year olds 16-year olds
    30 minutes 66
    68
    59
    72
    46    		 74
    71
    67
    82
    76
    60 minutes 69
    61
    69
    73
    61    		 95
    92
    95
    98
    94

  18. Define "Three-way interaction"
  19. Define interaction in terms of simple effects.
  20. Plot an interaction for an A(2) x B(2) design in which the effect of B is greater at A1 than it is at A2. The dependent variable is "Number correct." Make sure to label both axes.
  21. Following are two graphs of population means for 2 x 3 designs. For each graph, indicate which effect(s) (A, B, or A x B) are nonzero.

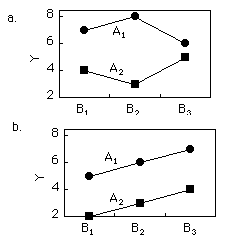
  22. The following data are from an A(2) x B(4) factorial design.
    23.   B1 B2 B3 B4
    A1 1
    3
    4
    5    		 2
    2
    4
    5    		 3
    4
    2
    6

    4
    5
    6
    8
    A2 1
    1
    2
    2    		 2
    3
    2
    4    		 4
    6
    7
    8    		 8
    9
    9
    8
    1. Compute an analysis of variance.
    2. Test differences among the four levels of B using the Bonferroni correction.
    3. Test the linear component of trend for the effect of B.
    4. Plot the interaction.
    5. Describe the interaction in words.


  23. Why are within-subjects designs usually more powerful than between-subjects design?

  24. What source of variation is found in an ANOVA summary table for a within-subjects design that is not in in an ANOVA summary table for a between-subjects design. What happens to this source of variation in a between-subjects design?

  25. The following data contain three scores from each of five subjects. The three scores per subject are their scores on three trials of a memory task.

    4 6 7
    3 7 7
    2 8 5
    1 4 7
    4 6 9

    1. Compute an ANOVA
    2. Test all pairwise differences between means using the Bonferroni test at the .01 level.
    3. Test the linear and quadratic components of trend for these data.

  26. Give the source and df columns of the ANOVA summary table for the following experiments:
    1. Twenty two subjects are each tested on a simple reaction time task and on a choice reaction time task.
    2. Twelve male and 12 female subjects are each tested under three levels
      of drug dosage: 0 mg, 10 mg, and 20 mg.
    3. Twenty subjects are tested on a motor learning task for three trials a day for two days.
    4. An experiment is conducted in which depressed people are either assigned to a drug therapy group, a behavioral therapy group, or a control group. Ten subjects are assigned to each group. The level of measured once a month for four months.
    27. Questions from Case Studies:


    The following question is from the Stroop Interference case study.

     

  27. The dataset has the scores (times) for males and females on each of three tasks.

    a. Do a Gender (2) x Task (3) analysis of variance.
    b. Plot the interaction.

    28. The following question is from the ADHD Treatment case study.

  28. The data has four scores per subject.
    1. Is the design between-subjects or within-subjects?
    2. Create an ANOVA summary table.

      3. The following question is from the Angry Moods case study.

  29. Using the Anger Expression Index as the dependent variable, perform a 2x2 ANOVA with gender and sports participation as the two factors. Do athletes and non-athletes differ significantly in how much anger they express? Do the genders differ significantly in Anger Expression Index? Is the effect of sports participation significantly different for the two genders?


    30. The following question is from the Weapons and Aggression case study.

  30. Compute a 2x2 ANOVA on this data with the following two factors: prime type (was the first word a weapon or not?) and word type (was the second word aggressive or non-aggressive?). Consider carefully whether the variables are between-subject or within-subects variables.

    31. The following question is from the Smiles and Leniency case study.

  31. Compute the ANOVA summary table.

     

     

     

     

Please answer the questions:
correct feedback