Home

  1. Introduction
  2. Graphing Distributions

  3. Summarizing Distributions
    1. Contents
      Standard
    2. Central Tendency
      Standard         Video
    3. What is Central Tendency
      Standard         Video
    4. Measures of Central Tendency
      Standard         Video
    5. Balance Scale Simulation
      Standard         Video
    6. Absolute Differences Simulation
      Standard
    7. Squared Differences Simulation
      Standard
    8. Median and Mean
      Standard         Video
    9. Mean and Median Demo
      Standard
    10. Additional Measures
      Standard         Video
    11. Comparing Measures
      Standard         Video
    12. Variability
      Standard         Video
    13. Measures of Variability
      Standard         Video
    14. Variability Demo
      Standard
    15. Estimating Variance Simulation
      Standard
    16. Shapes of Distributions
      Standard  
    17. Comparing Distributions Demo
      Standard
    18. Effects of Linear Transformations
      Standard         Video
    19. Variance Sum Law I
      Standard         Video
    20. Statistical Literacy
      Standard
    21. Exercises
      Standard

  4. Describing Bivariate Data
  5. Probability
  6. Research Design
  7. Normal Distribution
  8. Advanced Graphs
  9. Sampling Distributions
  10. Estimation
  11. Logic of Hypothesis Testing
  12. Tests of Means
  13. Power
  14. Regression
  15. Analysis of Variance
  16. Transformations
  17. Chi Square
  18. Distribution Free Tests
  19. Effect Size
  20. Case Studies
  21. Calculators
  22. Glossary
 

Shapes of Distributions

Author(s)

David M. Lane

Prerequisites

Distributions, Measures of Central Tendency, Variability

Learning Objectives
  1. Compute skew using two different formulas
  2. Compute kurtosis

We saw in the section on distributions in Chapter 1 that shapes of distributions can differ in skew and/or kurtosis. This section presents numerical indexes of these two measures of shape.

Skew

Figure 1 shows a distribution with a very large positive skew. Recall that distributions with positive skew have tails that extend to the right.

Figure 1. A distribution with a very large positive skew. This histogram shows the salaries of major league baseball players (in thousands of dollars).

Distributions with positive skew normally have larger means than medians. The mean and median of the baseball salaries shown in Figure 1 are $1,183,417 and $500,000 respectively. Thus, for this highly-skewed distribution, the mean is more than twice as high as the median. The relationship between skew and the relative size of the mean and median led the statistician Pearson to propose the following simple and convenient numerical index of skew:

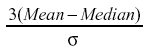
The standard deviation of the baseball salaries is 1,390,922. Therefore, Pearson's measure of skew for this distribution is 3(1,183,417 - 500,000)/1,390,922 = 1.47.

Just as there are several measures of central tendency, there is more than one measure of skew. Although Pearson's measure is a good one, the following measure is more commonly used. It is sometimes referred to as the third moment about the mean.

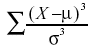
Kurtosis

The following measure of kurtosis is similar to the definition of skew. The value "3" is subtracted to define "no kurtosis" as the kurtosis of a normal distribution. Otherwise, a normal distribution would have a kurtosis of 3.

 

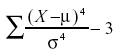
Please answer the questions:
correct feedback