Home

  1. Introduction
  2. Graphing Distributions
  3. Summarizing Distributions
  4. Describing Bivariate Data
  5. Probability
  6. Research Design
  7. Normal Distribution
  8. Advanced Graphs
  9. Sampling Distributions
  10. Estimation
  11. Logic of Hypothesis Testing
  12. Tests of Means
  13. Power
  14. Regression
  15. Analysis of Variance
  16. Transformations
  17. Chi Square
  18. Distribution Free Tests
  19. Effect Size

  20. Case Studies
    1. Contents
      Standard
    2. Angry Moods
      Standard
    3. Flatulence
      Standard
    4. Physicians Reactions
      Standard
    5. Teacher Ratings
      Standard
    6. Diet and Health
      Standard
    7. Smiles and Leniency
      Standard
    8. Animal Research
      Standard
    9. ADHD Treatment
      Standard
    10. Weapons and Aggression
      Standard
    11. SAT and College GPA
      Standard
    12. Stereograms
      Standard
    13. Driving
      Standard
    14. Stroop Interference
      Standard
    15. TV Violence
      Standard
    16. Obesity and Bias
      Standard
    17. Shaking and Stirring Martinis
      Standard
    18. Adolescent Lifestyle Choices
      Standard
    19. Chocolate and Body Weight
      Standard
    20. Bedroom TV and Hispanic Children
      Standard
    21. Weight and Sleep Apnea
      Standard
    22. Misusing SEM
      Standard
    23. School Gardens and Vegetable Consumption
      Standard
    24. TV and Hypertension
      Standard
    25. Dietary Supplements
      Standard
    26. Young People and Binge Drinking
      Standard
    27. Sugar Consumption in the US Diet
      Standard
    28. Nutrition Information Sources and Older Adults
      Standard
    29. Mind Set Exercise and the Placebo Effect
      Standard
    30. Predicting Present and Future Affect
      Standard
    31. Exercise and Memory
      Standard  
    32. Parental Recognition of Child Obesity
      Standard
    33. Educational Attainment and Racial, Ethnic, and Gender Disparity
      Standard

  21. Calculators
  22. Glossary
  Exercise and Memory

Research conducted by: M. E. Hopkins, F. C. Davis, M. R. Van Tieghem, P. J. Whalen, and D. J. Bucci

Case study prepared by: Robert F. Houser and Georgette Baghdady

Overview
Physical exercise has many beneficial effects on physiological processes, including those that affect cognition and memory. Exercise increases brain-derived neurotrophic factor (BDNF), which is a protein found in the learning and memory centers of the brain where it supports nerve cell survival and the growth of new neurons and neuronal connections. A polymorphism of BDNF (a variant genotype) alters the release of BDNF during exercise. The researchers of this study sought to compare the effects of a single bout of exercise versus a 4-week exercise regimen on cognition and memory and to determine if BDNF genotype influences the intensity of those effects of exercise.

Questions to Answer
How do regular exercise and/or an acute bout of exercise affect cognitive memory? Does type of BDNF genotype (Val/Val or Met carrier) mediate the effect of exercise on memory? How do we calculate a one-way ANOVA by hand and how do different post-hoc tests compare?

Design Issues
The group sample sizes are small, perhaps limiting the power to detect significant differences between the four exercise/control groups.

Descriptions of Variables
Variable Description
Group 0W-: sedentary group
0W+: sedentary group with one bout of exercise at least 2 hours before Visit 2
4W-: regularly exercising group
4W+: regularly exercising group with a bout of exercise at least 2 hours before Visit 2

Accuracy

 The percentage of objects each group accurately identified as old or new when performing the novel object recognition task during each study visit
Difference score Accuracy achieved by the subject in the novel object recognition task during Visit 2 minus accuracy during Visit 1, in percent
BDNF genotype Whether a subject's BDNF genotype is Val/Val or Met carrier (Val/Met and Met/Met)


References

Hopkins, M. E., Davis, F. C., Van Tieghem, M. R., Whalen, P. J., Bucci, D. J. (2012). Differential effects of acute and regular physical exercise on cognition and affect. Neuroscience, 215, 59-68.

 

Links

How Exercise Affects the Brain: Age and Genetics Play a Role
BDNF


Exercises
The following table contains the mean, standard error of the mean (SEM), and sample size for the difference in scores between Visit 2 and Visit 1 (Visit 2 score – Visit 1 score) on the object recognition memory test for each of the three exercise groups and control group. The data are illustrated in Figure 3B of the article as a bar graph.

Group Mean SEM SD Sample size (n)
0W-
(sedentary group)		 -4.50 2.08     13
4W-
(regularly exercising group)		 -4.90 1.60   14
4W+
(regularly exercising group with a bout of exercise at least two hours before Visit 2		 2.42 2.36   12
0W+
(sedentary group with one bout of exercise at least two hours before Visit 2)		 -2.50 1.50   15
  1. Calculate the standard deviations (SD) for the four groups and record the values you obtain in the table. Recall that SEM = SD/√n. Report SD values to six decimal places.

    Do the mean difference scores for accuracy in the novel object recognition task (i.e., accuracy during Visit 2 minus accuracy during Visit 1) differ significantly among the four groups (0W-, 4W-, 4W+, 0W+)? To answer this question, you first need to perform a one-way ANOVA, which you will do by hand since we do not have the raw data.
  2. What is the null hypothesis for the one-way ANOVA? What is the alternative hypothesis?

    The following table contains all of the formulas you will need to calculate the F statistic of a one-way ANOVA.

    formulas'
  3. First, you need to calculate the Grand Mean, which is used in the calculation of the Sum of Squares Between. The Grand Mean equals the weighted average of the four group means using the respective group sample sizes as the weights. Write out the equation and report the value.
  4. Use the formulas presented in the above table to calculate the F statistic for comparing the means of the four groups (0W-, 4W-, 4W+, 0W+). Fill in the table below with the values you obtain.

    Source Sum of Squares (SS) Degrees of Freedom (df) Mean Square (MS) F
    Between        
    Within        
    Total        

  5. The degrees of freedom for the F statistic is the pair of numbers dfB (from the numerator) and dfW (from the denominator). Use the online calculator that computes the probability for the F distribution.
  6. In the article, the authors used independent samples t tests. Perform independent samples t tests based on p<0.05 for the six possible comparisons shown in the table below and fill in the values you obtain. Interpret your results based on what the group notations represent. For example, comparing 4W- versus 4W+ determines whether or not a bout of exercise a few hours before engaging in a task requiring cognition and memory improves performance on that task in regularly-exercising individuals.

    Pairwise comparison t statistic p value
    0W-, 4W-    
    0W-, 4W+    
    0W-, 0W+    
    4W-, 4W+    
    4W-, 0W+    
    4W+, 0W+    

  7. Now apply the Bonferroni correction. Since you performed six pairwise comparisons, two means will be considered statistically significantly different if the p value is less than 0.05 / 6 = 0.0083. Based on the Bonferroni correction, are the pairwise comparisons that were statistically significant in Exercise #6 still statistically significant? If yes, which ones?
  8. The Tukey HSD test is the most commonly used post-hoc test following an ANOVA. Perform the Tukey HSD test for the pairs of means that you found to be statistically significant in Exercise #6. The formula and link for the test's Q statistic are given below.

    The Q statistic for the Tukey HSD test is computed as follows:
    Q = (Mi – (Mj) / [√(MSW / harmonic mean)],
    where
    Mi is one mean,
    Mj is the other mean,
    MSW is the value you calculated in Exercise #4,
    and the harmonic mean = 2 / (1/ni + 1/nj), where the n's are the sample sizes in the pairwise comparison

    Use the Studentized Range Distribution to obtain the p value. Based on the Tukey tests, are the pairwise comparisons that were statistically significant in Exercise #6 still statistically significant? If so, which ones?

  9. Which of the post-hoc approaches was/were the most conservative with these data?
  10. Determine the effect size attributable to groups, that is, the proportion of the variance among subjects' difference scores that is attributable to the experimental group they were in. Use the formula presented in the chapter on effect size:
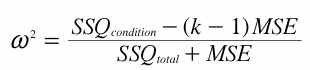    formula. Interpret your result. Figure 3C in the article shows a bar graph of the difference scores in each of the four groups (0W-, 4W-, 4W+, 0W+) stratified by the subjects' BDNF genotype, either Val/Val or Met carrier.
  11. For the 4W+ group, perform an independent samples t test based on p<0.05 to compare the mean difference scores between subjects with Val/Val genotype and those who are Met carriers. What do you conclude?
    GROUP VAL/VAL MET CARRIER
      Mean SEM SD n Mean SEM SD n
    4W+ 7.5 2.97 7.2749 6 -2.7 2.3 5.6338 6

  12. How did the researchers formulate their predictions about exercise and memory in humans?
  13. What physiological mechanism(s) do the authors propose that support their findings?